Trisections of the Area of Triangles
By: Lacy Carter
Problem 1
Given a triangle ABC, find a point D such that line segments AD, BD, and CD trisect the area of the triangle into three regions with equal areas.
△ABC should look similar to the triangle depicted below.
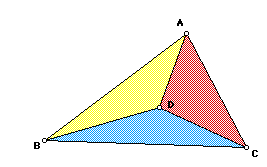
First, I let D be the point of concurrency of the angle bisectors. The triangle was trisected into three smaller triangles and it looked like the triangle we were trying to mimic.
However, the areas of the three triangles were not the same.
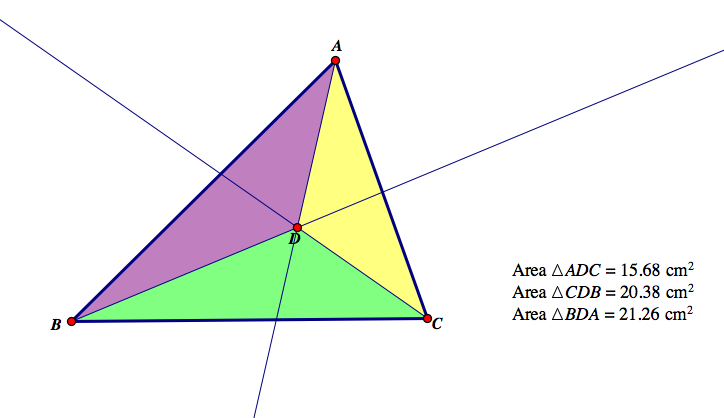
Next, I let D be the point of concurrency of the medians of the triangle. We see that the areas of the three smaller triangles are equal as desired.
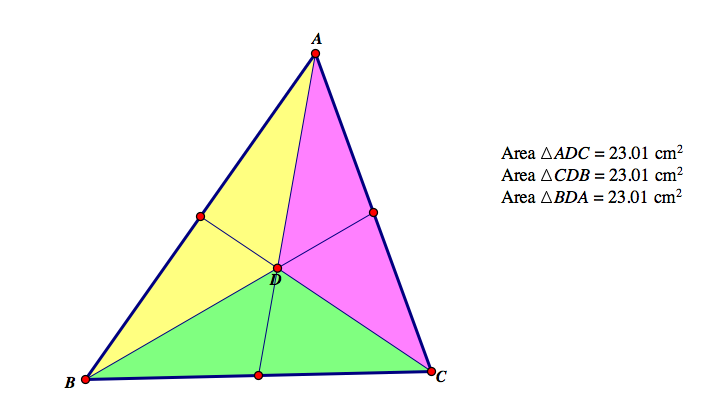
The Centroid of a triangle is the point of concurrency of the three medians.
Recall, a median of a triangle is the segment from a vertex to the midpoint of the opposite side. The medians divide the triangle into six smaller triangles.
We want to show that △ADB, △ADC, and △BDC have equal areas.
Lets start by showing that the six triangles formed by the medians have the same area.
We found that 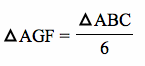
The area of ΔAGF is ⅙ of the area of ΔABC.
The areas of the remaining 5 remaining triangles are each equal to ⅙ the area of ΔABC, proving that the medians divide the triangle into 6 smaller triangles of equal area.
△ADB, △ADC, and △BDC are each made up of two of these smaller triangles.
Since these 6 small triangles have equal area, we can conclude that △ADB, △ADC, and △BDC have equal areas as well.
Problem 2
Given a triangle ABC, and given a point E, find points B' and C' such that line segments AE, B'E, and C'E trisect the area of the triangle into three regions with equal areas.
△ABC should look similar to the triangle depicted below.
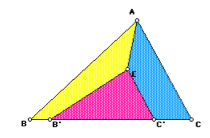
Base Case:
First, let E be located on vertex A. If EB' and EC' trisect the area of the △ABC, then B' and C' must be the trisection points of BC.
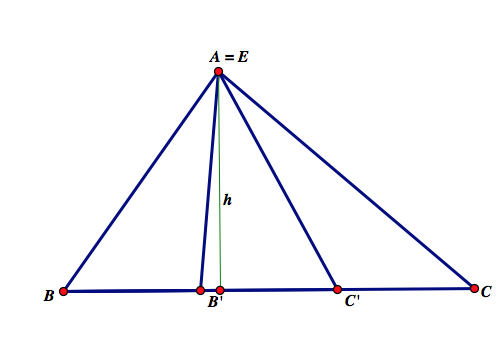
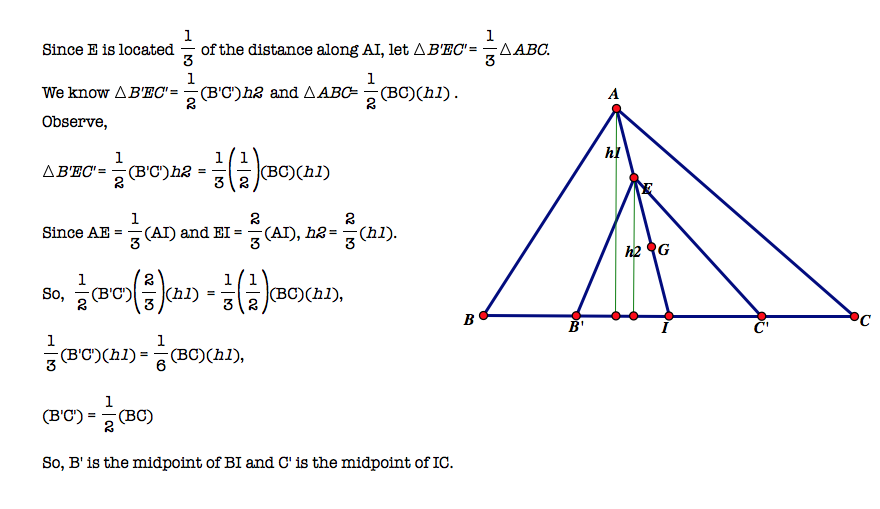
From Problem 1 and our explorations of the base case, we can conclude that point E must lie on a median of △ABC between one of the triangle's vertexs and its centroid, G. So point E cannot lie closer than (1/3) the length of median AI (point I is identified in the picture below) to side BC. If point E was closer than this, the triangle would not be trisected into three region with equal areas.
Let AE be (1/3) the distance from a vertex, to the midpoint of the opposite side.
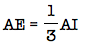
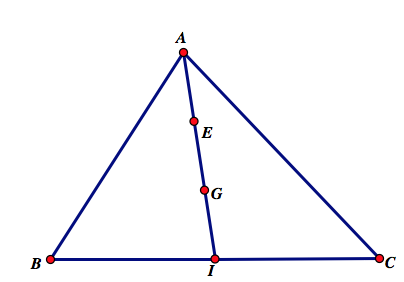
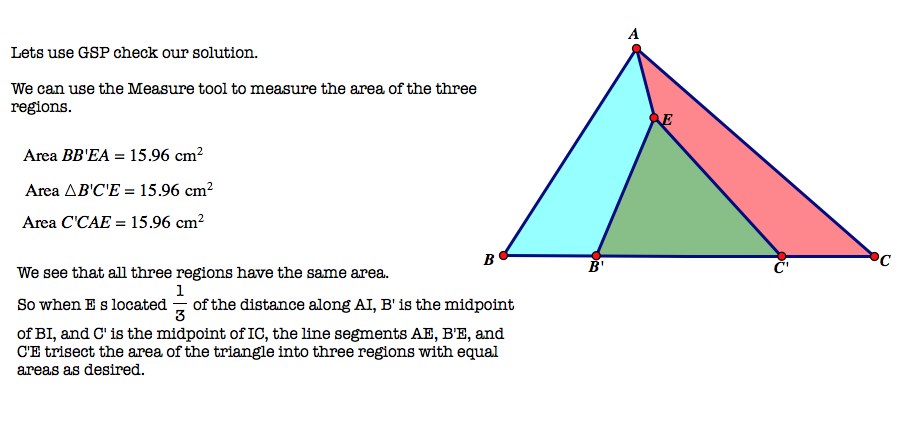
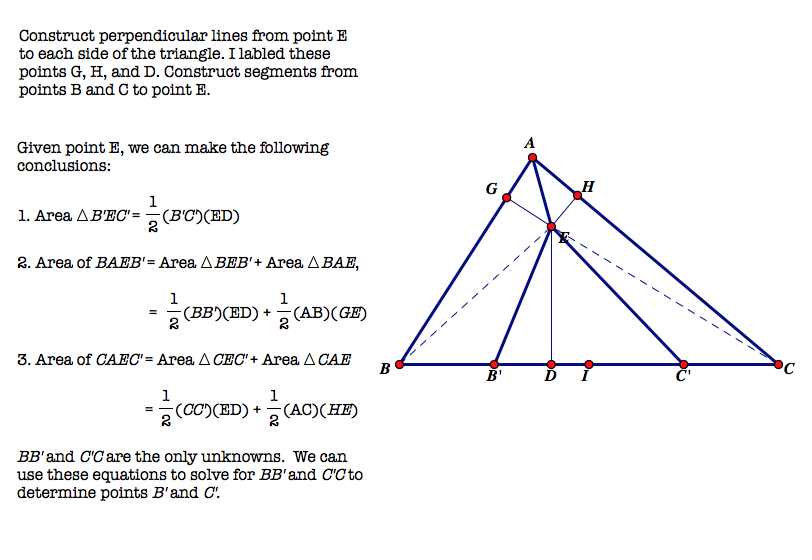
First, I found the centroid, G, of the triangle and then found the midpoint of AG. E is the midpoint of AG.
Problem 3
Given a triangle ABC. Construct two line segments parallel to the base BC to divide the triangle into three regions with equal areas.
△ABC should look similar to the triangle depicted below.

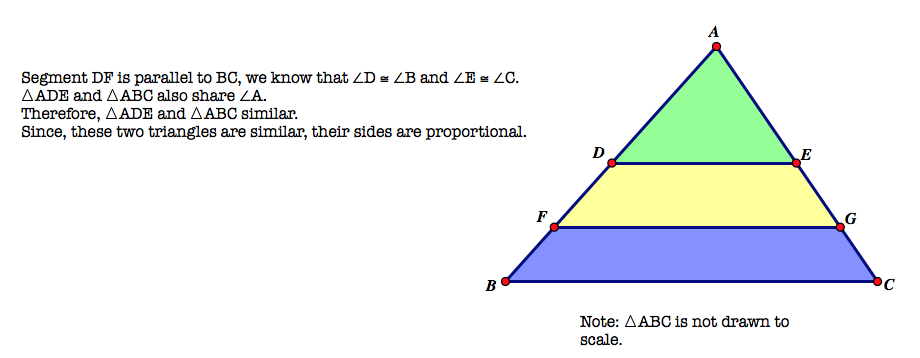
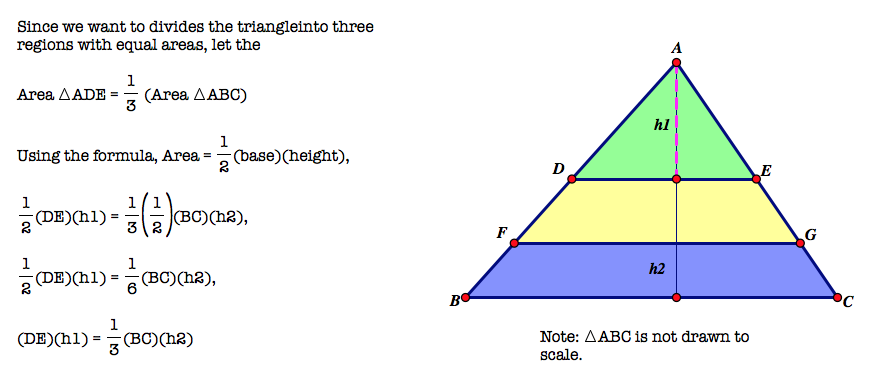

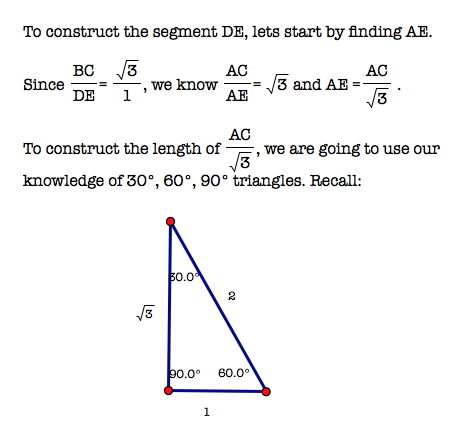
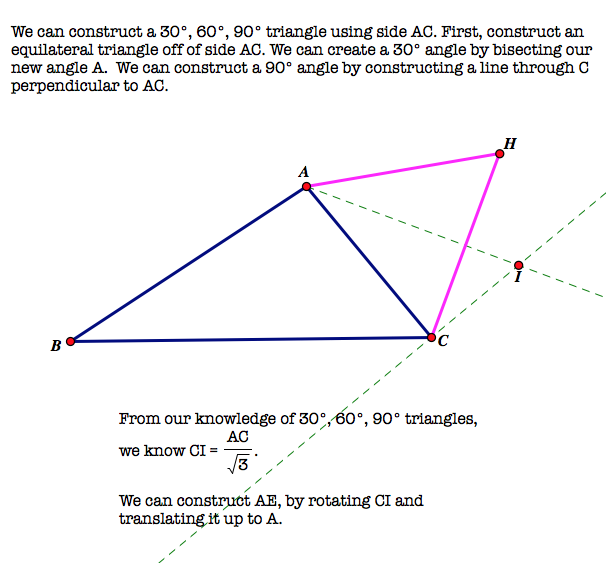
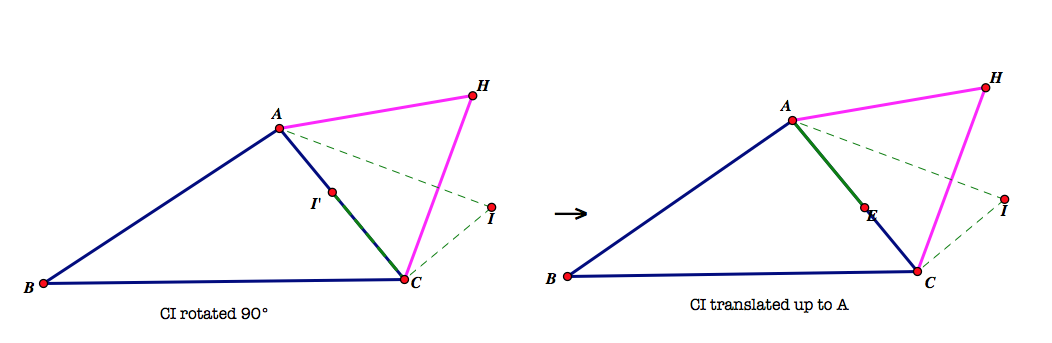
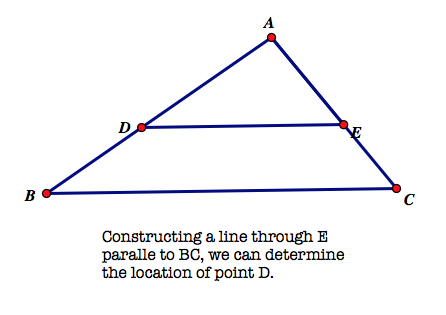
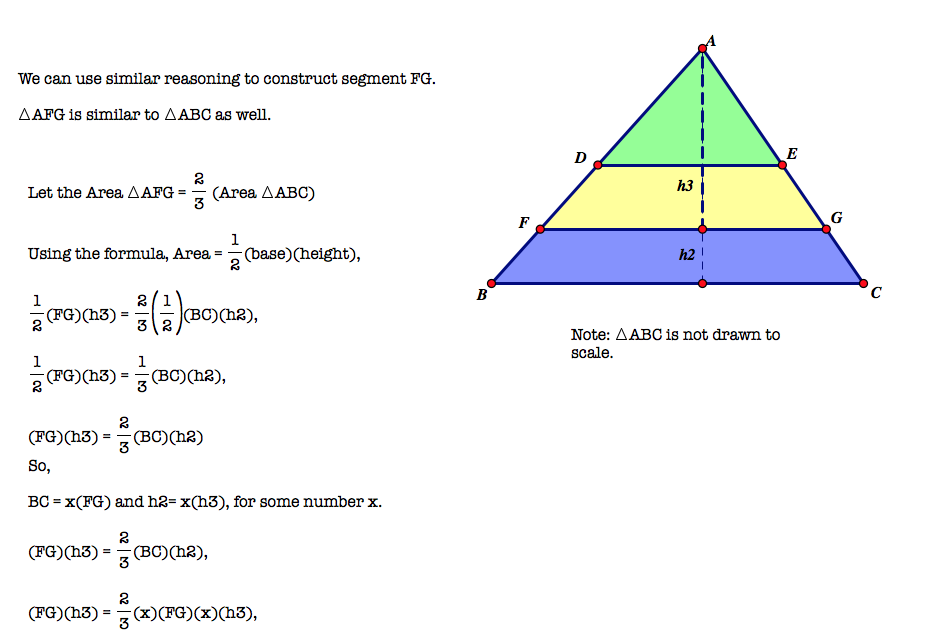

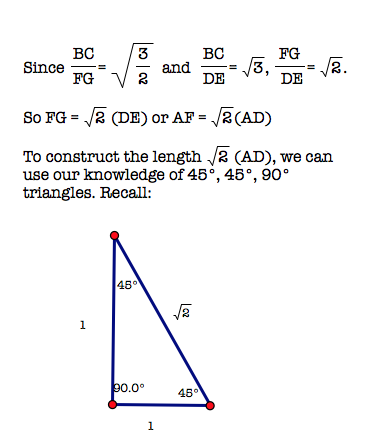
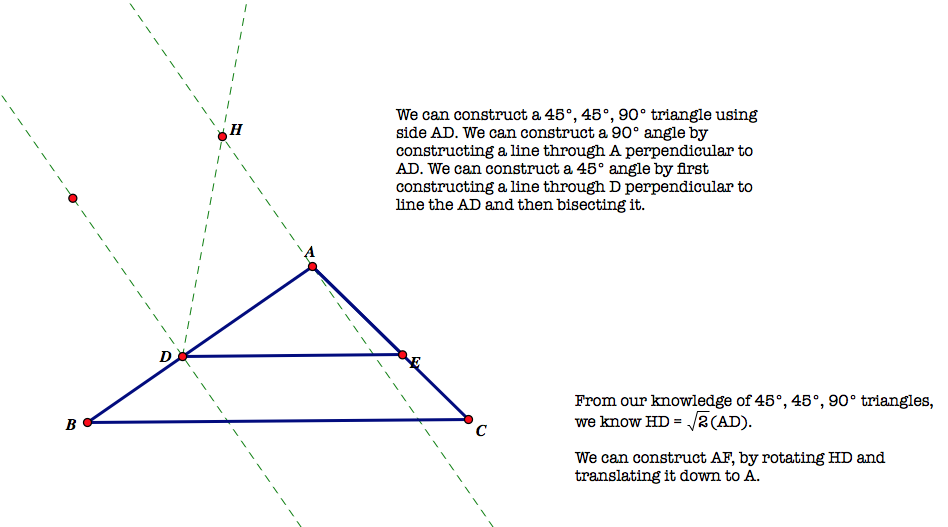
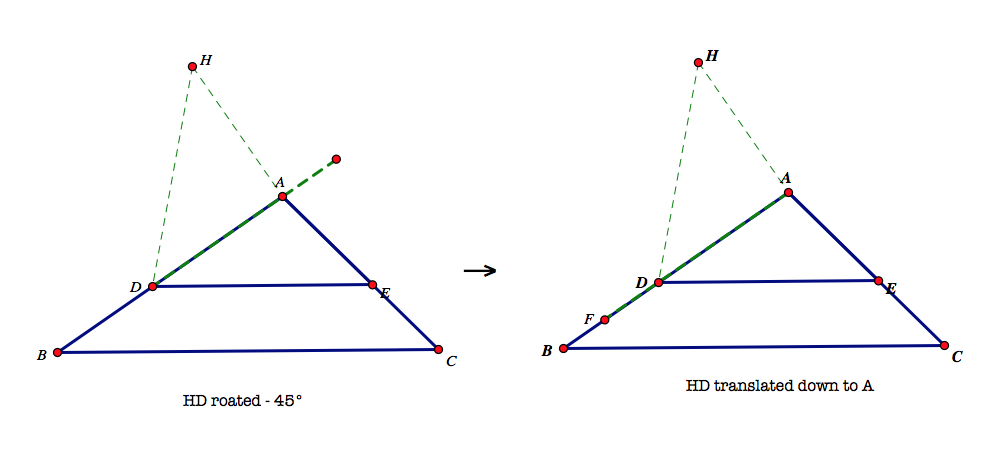
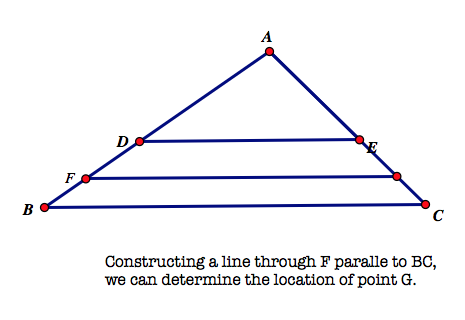
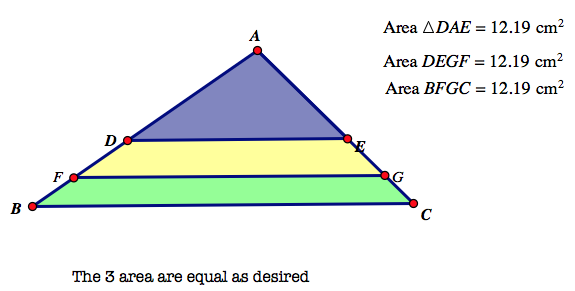
Now, prove that the construction divides the triangle into three regions of equal area.

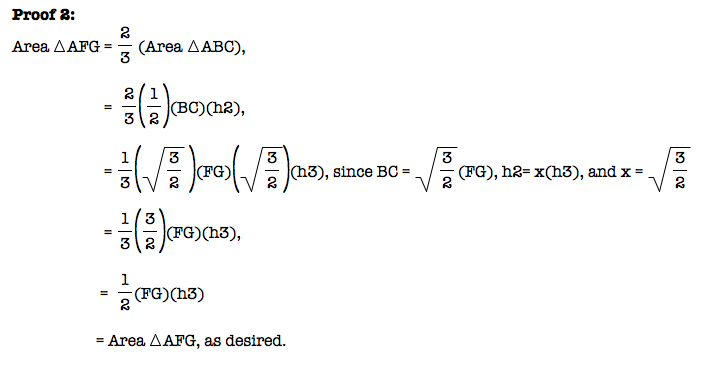
So, we have shown that the Area △ADE = (1/3) (Area △ABC) and the Area △AFG = (2/3) (Area △ABC).
Therefore, △ADE and quadrilaterals DEGF and BFGC each have an area eqaul to (1/3) the Area of △ABC as desired.

